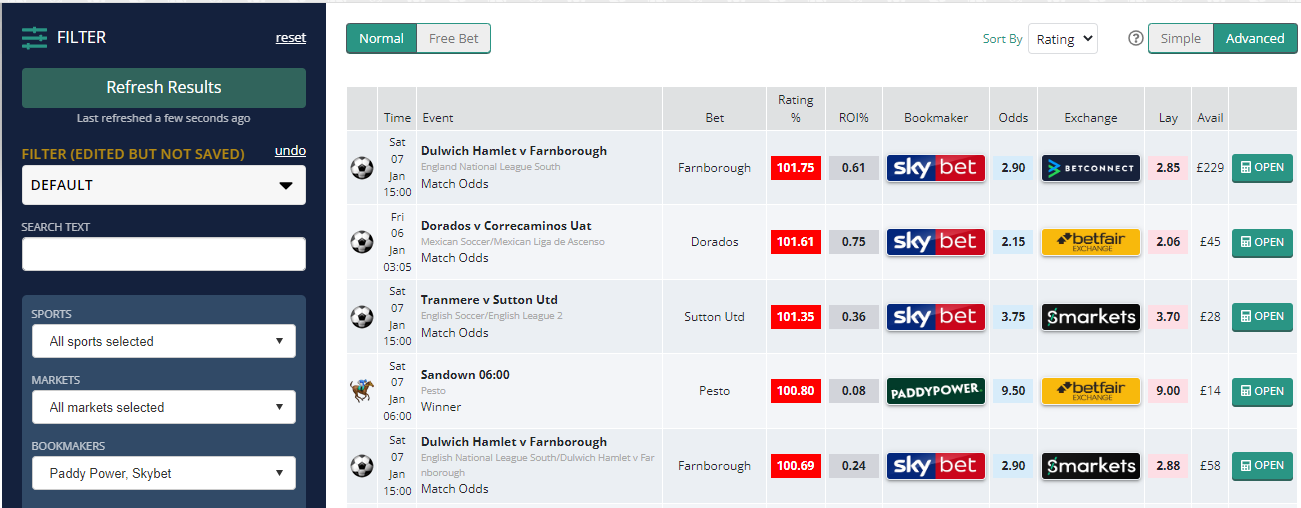
If you’ve heard professional gamblers talking about “positive EV” or “+EV” but didn’t understand, then this explanation is for you.
In simple terms, we’re explaining what Positive EV means in betting…
What is +EV in Betting?
In betting, positive expected value (EV) refers to a situation where the potential payout from a bet is higher than the risk involved. In other words, positive EV means that the bet is expected to be profitable in the long run as variance plays out.
To understand positive EV, it’s important to know about the concept of probability. Probability is a measure of how likely an event is to occur. For example, if a coin is flipped, the probability of it landing on heads is 50%. That’s a fact!
In betting, probability is used to calculate the odds of an event occurring. Odds can be expressed in several ways, such as fractional odds (e.g. 3/1), decimal odds (e.g. 4.0), or American odds (e.g. +300).
To calculate the expected value of a bet, you need to determine the probability of the event occurring, and then multiply that probability by the payout if the event does occur. For example, if you bet £100 on a football game with odds of 6/4 in fractions, decimal odds of 2.5, and American +150, the expected value of the bet is calculated as follows:
(Probability of winning * Payout) – (Probability of losing * Risk)
=(1 / (1 + 150)) * 250 – (1 – (1 / (1 + 150))) * 100
= 0.4 * 250 – 0.6 * 100
= 100 – 60
= £40
In this case, the expected value of the bet is positive. It means that the bet is expected to be profitable in the long run. However, it’s important to note that expected value is a long-term concept, and it doesn’t guarantee that you’ll win every individual bet. In the short term, anything can happen, and you could still lose even if the expected value of the bet is positive.
One way to guarantee a positive expected value each day is through betting arbitrage or matched betting with services like Odds Monkey.
To give another example, suppose you’re considering betting on a roulette wheel. The probability of winning on a single number bet is 2.7%, and the payout is 35 to 1. The expected value of this bet is calculated as follows:
(Probability of winning * Payout) – (Probability of losing * Risk)
=(0.027 * 35) – (0.973 * 1)
= 0.94 – 0.973
= -0.033
In this case, the expected value of the bet is negative, meaning that the bet is expected to be unprofitable in the long run. Of course, it’s still possible to win individual bets, but over time, the house edge will likely lead to a loss.
It’s important to note that expected value is just one factor to consider when making a betting decision. There are other factors, such as risk tolerance, personal preferences, and the potential for excitement and enjoyment, that can also influence the decision to place a bet.
In conclusion, positive expected value in betting refers to a situation where the potential payout from a bet is higher than the risk involved. It’s a long-term concept that can be used to help make informed betting decisions, but it doesn’t guarantee success in every individual bet. Short-term variance still applies.